

Linear-Quadratic Systems of Equations Notes. Write the general formula for the following sequence 6, 18, 54, 162, in. Geometric Sequences and Finding the nth Term Given the. Answer: an 3(2)n 1 a10 1, 536 The terms between given terms of a geometric sequence are called geometric means21. an a1rn - 1 where a1 is the first term and r is the common ratio. So the rst ten terms of the sequence are: 1,1,2,3,5,8,13,21,34,55 This sequence continues forever.


To continue the sequence, we look for the previous two terms and add them together. The fourth number in the sequence will be 1 + 2 3 and the fth number is 2+3 5.
#Algebra 2 geometric sequences formula series
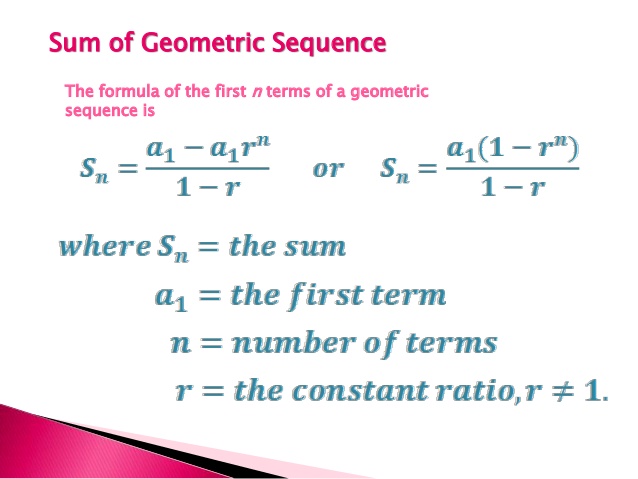
1: Sequences and Series Arithmetic/Geometric SeriesThe International. numbers of the sequence be 1 and let the third number be 1 + 1 2. The common ratio is multiplied by the first term once to find the second term, twice to find the third term, three times to find the fourth term, and so on. Algebra 2 course, and also introduces the concept of sequences. Algebra 2 Comparing Arithmetic and Geometric Sequences Formula Worksheets, Algebra. What Is The Formula For A Geometric Sequence. Mathematics: applications and interpretation formula booklet 2. step-by-step solutions to geometric sequence. In geometric sequences, to get from one term to another, you multiply, not add. calculator, prentice hall algebra 2 powerpoint, math questions logarithms. Therefore, a convergent geometric series 24 is an infinite geometric series where \(|r| < 1\) its sum can be calculated using the formula:īegin by identifying the repeating digits to the right of the decimal and rewrite it as a geometric progression.\] Geometric sequences differ from arithmetic sequences.


 0 kommentar(er)
0 kommentar(er)
